LaTeX templates and examples — Math

Calculating the value of Ck ∈ {1, ∞} class of smoothness real-valued function's derivative in point of R+ in radius of convergence of its Taylor polynomial (or series), applying an analog of Newton's binomial theorem and q-difference operator. (P,q)-power difference introduced in section 5. Additionally, by means of Newton's interpolation formula, the discrete analog of Taylor series, interpolation using q-difference and p,q-power difference is shown.

In this paper, we derive and prove, by means of Binomial theorem and Faulhaber's formula, the following identity between $m$-order polynomials in \(T\) \(\sum_{k=1}^{\ell}\sum_{j=0}^m A_{m,j}k^j(T-k)^j=\sum_{k=0}^{m}(-1)^{m-k}U_m(\ell,k)\cdot T^k=T^{2m+1}, \ \ell=T\in\mathbb{N}.\)

The main aim of this paper to establish the relations between forward, backward and central finite (divided) differences (that is discrete analog of the derivative) and partial & ordinary high-order derivatives of the polynomials.

Cour 1 ere AS

Primality Tests and Factoring Algorithms

Two Snow Plows Problem

"(Infinite) series are the invention of the devil, by using them, on may draw any conclusion he pleases, and that is why these series have produced so many fallacies and so many paradoxes." -Neils Hendrik Abel

Señales y Sistemas

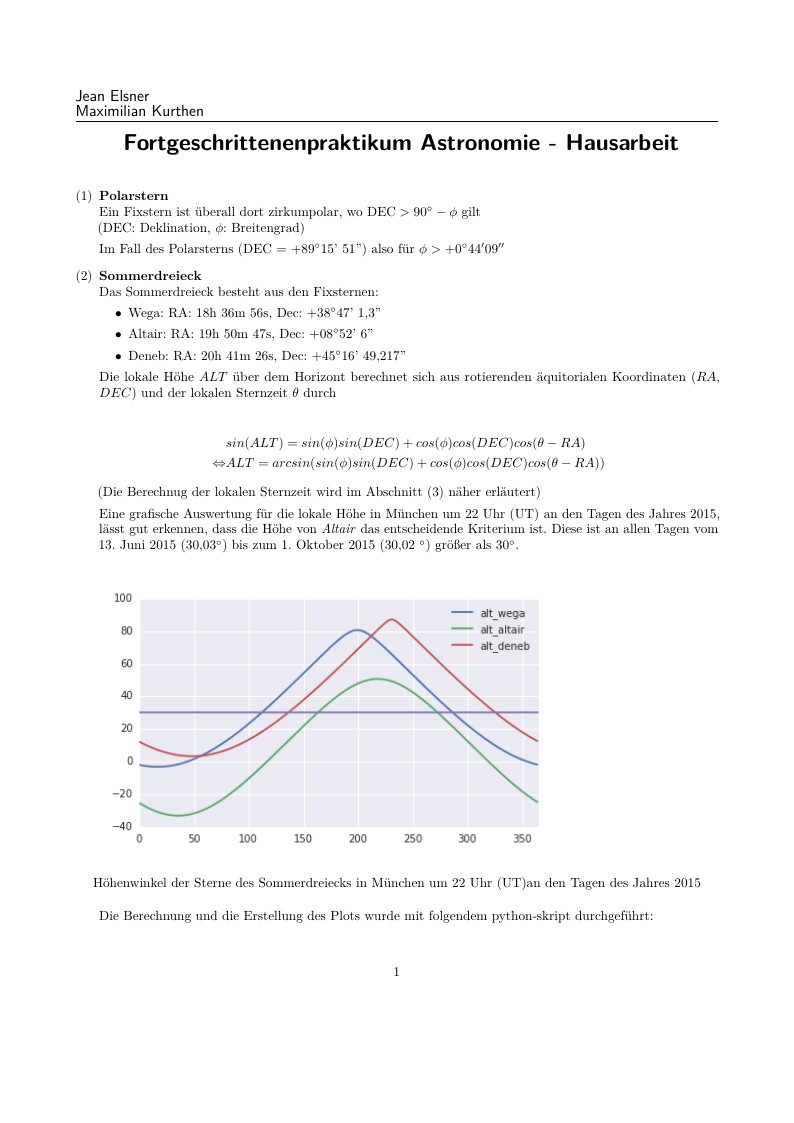
Fortgeschrittenenpraktikum Astronomie Hausarbeit an der Universitäts-Sternwarte München (LMU).